Electricity Generation
Summary
This section describes in detail the representing of the existing generation fleet and assumptions for new electric generation capacity options, including retrofits and conversions of existing technologies. Technology cost and performance assumptions are provided in the New Generation Capacity section, while additional details about specific technologies and parameters are provided in the subsequent sections.
Existing Fleet
Aggregation
Although the existing generation fleet includes thousands of units, computational tractability within the multi-region inter-temporal optimization framework requires aggregation. For each region, fossil units with similar attributes were aggregated into blocks based on the principle that all units in a given block would be dispatched similarly based on market conditions. Since the most important factor for determining dispatch order is energy cost, units within each fuel group were classified according to heat rate. Additionally, gas turbine units and single cycle steam units were treated separately from combined cycle units. The aggregation process was designed to be flexible so that different schemes can be applied depending on the objectives of the analysis. For example, for an analysis of the value of retrofitting existing coal units with environmental controls, the coal units were aggregated by region in terms of the cost of retrofitting, for a total of twelve blocks per region. The current default formulation uses four coal blocks, seven gas blocks, and one petroleum block per region to represent the existing fossil fleet.
For each aggregated capacity block, the values of relevant performance attributes, such as heat rate and variable operating costs, were determined by taking the capacity weighted average of the parameter of interest across units assigned to that capacity block. In addition, an age distribution was calculated for each block of existing capacity based on the online year of the constituent units. Combined with an assumption about total lifetime after which a unit must retire, this translates into a "survival fraction" across model time periods of the block's initial capacity endowment. This distribution effectively creates a set of vintages within each existing capacity block for the purposes of retirement schedule, although the cost and performance characteristics (and therefore dispatch) are the same across vintages.
Existing nuclear capacity is represented by a single block per region with a similar age distribution approach. However, rather than a single lifetime, a percentage of capacity in each region (amounting to roughly 75% nationwide) was assumed to receive 20-year license extensions, taking their total lifetimes to 80 years, while the remainder were limited to 60-year licenses.[1] Announced retirements are incorporated explicitly where this occurs earlier than in the above calculation. The model also assumes a limited amount of uprate capacity (roughly 2.1 GW nationally) that slightly increases the existing nuclear endowment in the first decade of the model's time horizon.
Existing renewable capacity is also represented by a single block per technology per region, with blocks representing onshore wind, utility PV, rooftop PV, concentrated solar, hydro, geothermal, and biomass.[2] Wind, solar, and geothermal units have limited lifetimes, typically around 30 years, with the option to repower the site with a new unit. Biomass units have a 60 year lifetime. The hydro block has unlimited lifetime. Because most hydro capacity takes advantage of finite special resources, such as a geographically advantageous dam location, it may reasonably be expected neither to expand nor phase out over time.
Table 2‑1 describes the classification of existing generation.
| Generation | Number of Classes | Lifetime (years) | 2015 Total Capacity (GW) |
|---|---|---|---|
| Coal | 4 (based on heat rate) | 70 | 266 |
| Natural Gas (combined cycle) | 3 (based on heat rate) | 40 | 237 |
| Natural Gas (gas turbine) | 3 (based on heat rate) | 60 | 128 |
| Natural Gas (single cycle steam) | 1 | 70 | 75 |
| Petroleum | 1 | 70 | 37 |
| Nuclear | 1 | 60-80 | 98 |
| Hydro (incl. pumped storage facilities) | 1 | Indefinite | 79 |
| Wind | 1 | 25 | 76 |
| Geothermal | 1 | 30 | 3 |
| Solar (PV and Concentrated) | 1 | 30 | 31 |
| Biomass and Other | 1 | 60 | 18 |
A dataset on the existing electricity generating fleet was procured from Energy Velocity LLC based on data reported on Form EIA-860 (Annual Electric Generator Report) as of December 2015. This dataset characterizes the existing fleet at the unit level in terms of installed capacity, average heat rate, fuel costs, variable and fixed O&M costs, and unit age (online year). Additional data on emissions rates for SOx and NOx and announced retirement dates are procured from Energy Velocity LLC as of early 2019. These characteristics, with the exception of fuel costs, are held constant across the model time horizon. To enable a single national price for the various fuels that varies over time and scenario while also preserving the observed heterogeneity in realized costs, differences in fuel costs across units are represented relative to the (time-dependent) national average, and these differences themselves are assumed to diminish over time.
Availability Factors
Availability factors were assigned to existing capacity through a de-rating process to capture average outages. In practice, units have their full capacity available in most hours, but are unavailable at certain times due to scheduled and unscheduled maintenance. Planned downtime is typically scheduled seasonally to coincide with lower expected loads. Thus a flat de-rating of capacity will underestimate availability at peak times, when unit operators plan for full capacity and are affected only by stochastic failure events. On the other hand, ignoring scheduled outages will overestimate total availability and therefore generation, particularly in the case of coal. Ideally, an hourly or daily availability factor could be inferred from reported unit operation data, but there are no data at this level of resolution. The closest approximation in publicly available EIA data is a table of monthly generation totals by state, and hence region, for aggregated fuel groups (e.g. coal, gas, nuclear, etc.) in historic years.
For existing nuclear and biomass / other capacity, a monthly availability factor was calculated to satisfy the equation
capacity × availability × hours in the month = generation in the month
for the calibration year 2015. This simple calculation was based on the idea that these units are "in the money" (i.e. have infra-marginal dispatch cost) all the time and hence not subject to dispatch decisions. For nuclear, with its very low variable costs, this is usually literally true; for the small amounts of existing biomass capacity, it is likely not literally true but it is a convenient simplifying assumption that will not strongly affect results. The average availability of existing nuclear units in 2015 was 93%. After 2015, both existing and new nuclear units are assumed to have a similar monthly shape and availability factor.
For fossil units, this calculation must be modified to include only "hours in the money"—the availability factor is intended to represent the average percentage of the time full capacity is available, which is likely greater than its dispatched capacity factor for high-variable cost units.[3] Because the choice of availability factor affects dispatch decisions, which affects "hours in the money" for the various units, these factors cannot be directly observed. Based on inspection of monthly generation patterns and a series of calibration experiments, we apply exogenous availability factors for coal and combined cycle natural gas that peak in the summer and winter and have annual averages of around 75% and 85% respectively. Gas turbines are assumed to have 100% availability.
For geothermal, a similar approach is taken as for nuclear (matching capacity to monthly generation using all monthly hours). The availability factor of geothermal is assumed to increase over time up to a maximum of 80%.
Hydro and pumped storage must match monthly generation constraints but are allowed to dispatch within the month subject to a minimum generation constraint that approximates stream flow and reservoir constraints. Because base year monthly generation of hydro may reflect anomalous water availability conditions, we use the long-run average monthly pattern to constrain generation in the model projection periods. Hourly dispatch of hydro resources is estimated in between model iterations with residual load serving as a proxy for the system price. This ensures that hydro dispatch coincides with peak residual load to the extent possible within the resource constraints. The resulting hourly profile of hydro dispatch is translated to the dynamic model via the weighted representative hours.
Availability factors are assigned to new thermal units based on the best-performing existing units.
For wind and solar power generators (existing and new), variability coefficients are based on hourly resource data as discussed in Renewable Resources.
New Generation Capacity
US-REGEN model assumptions for the costs and efficiency of candidate new generation technologies over time are shown in the chart and table below. Use the buttons to choose a parameter to compare. Descriptions of each technology can be found under the "Data Definitions" panel, below. All costs are shown in year 2015 dollars. Note that this table reflects the assumptions used in the model for the recently published LCRI Net-Zero 2050 Study. EPRI is continuously refining its technology cost and performance assumptions based on ongoing research and analysis.
Measures Electricity Generation TechnologyData Definitions
Retrofits and Conversions
US-REGEN includes the option to make retrofit investments to convert certain types of capacity to a different type. Eligible conversions include:
- retrofits of existing coal capacity to use natural gas or biomass (either 5% co-firing or 100% conversion);
- retrofits to add carbon capture and storage to existing coal and natural gas capacity; and
- conversions of new gas-fired capacity to use hydrogen in some future year.
These transformations incur a one-time capital cost and can result in capacity degradation and changes in operating parameters including variable costs, heat rates, and emission rates. Operating characteristics of converted capacity are expressed relative to the associated base (i.e. pre-retrofit) plant. In addition, most retrofits result in a capacity penalty, which is at least as large as the heat rate penalty (since such retrofits do not expand the thermal capacity of the plant) and may be larger. Retrofits and conversions do not change the age profile of the underlying capacity. For carbon capture retrofits for existing coal and gas, eligibility is limited to newer, larger plants. Table 2 outlines the assumed costs of each type of conversion, and the assumptions for the resulting changes in the plant's performance.
| Original Unit | Conversion | Eligibility | Capital Cost ($/kW) | Capacity Penalty | Efficiency Penalty |
|---|---|---|---|---|---|
| Existing Coal | → 90% carbon capture | <50 years old >100 MW <12,500 heat rate | 1750-2760 (varies by region and unit) | 22-24% (varies by region and unit) | 28-32% (varies by region and unit) |
| Existing Coal | → Gas | All | 165 | None | 0.05 |
| Existing Coal | → Bio | All | 1100 | 0.3 | 0.17 |
| Existing NGCC | → 90% carbon capture | <50 years old >200 MW <12,500 heat rate | 1450-2130 (varies by region and unit) | 16-18% (varies by region and unit) | 19-22% (varies by region and unit) |
| New NGCC | → Hydrogen | All new vintages | 175 | None | 0.05 |
| New Gas Turbines | → Hydrogen | All new vintages | 145 | None | 0.05 |
Emissions Rates
SO2 and NOx rates for existing units are constructed from two sources: unit-level EPA Continuous Emission Monitoring System (CEMS) data, as provided by ABB Energy Velocity, and EIA plant-level data for those units not reported in the CEMS dataset, typically small units. These emission rates are aggregated to the US-REGEN blocks using a capacity-weighted average. As these data are sourced from 2017 and 2018, not all the emission rates will reflect the addition of environmental controls to meet EPA regulations. Therefore, for the 2020 model time step and beyond, the emission rates are adjusted to ensure all units comply with the Mercury and Air Toxics Standards (MATS). SO2 rates are set equal to the minimum of the observed rate and the 0.2 lb/mmbtu MATS standard. NOx rates for existing units are held constant at the observed rate in 2018. REGEN includes a representation of the Cross-state Air Pollution Rule for both the annual fine particulate and the ozone season NOx requirements, but these constraints do not bind given assumed emission rates.
Emission rates for new units are based on data from EPRI's Generation Options report and historic emission rates from modern units and meet all current regulations for SO2 and NOx emission rates. New supercritical pulverized coal units without CCS are assumed to emit 0.075lb NOx/mmbtu, and 0.0858 lb SO2/mmbtu. New IGCC coal units with CCS are assumed to emit 0.051 lb NOx/mmbtu and 0.0017lb SO2/mmbtu, based on the best 12% of existing coal units. New combined cycle gas-fired units are assumed to emit 0.009 lb NOx/mmbtu.
CO2 emission rates are calculated from the average carbon content of the input fuel, multiplied by the average heat-rate of the unit in question. The carbon content of coal, refined petroleum, and natural gas respectively is set at 0.093, 0.073, and 0.054 tons CO2 per mmbtu. For units with carbon capture and storage, this rate is reduced by the assumed CO2 capture percentage.
Wind Technologies
Wind speed at hub height is translated into power output based on assumed power curves for a range of turbine technologies. The power curve for a wind turbine reflects key design parameters such as cut-in speed, efficiency, and most importantly, the ratio of nominal generator capacity to the swept area of the turbine blades, known as specific power or specific capacity (measured in W/m2). The lower the specific power, the lower the wind speed at which output reaches nameplate capacity, and hence the higher the capacity factor, as there are more hours with output at full capacity. Conventional wisdom has held that a lower specific power is more suitable for lower quality wind sites, because there are fewer hours at high speeds to justify a larger generator. However, as wind penetration has increased, the value of generation at high wind-speed moments is reduced due to the supply glut effect, placing a premium on power capture at lower speeds. The results it that lower specific power turbines are being deployed even for higher quality sites.
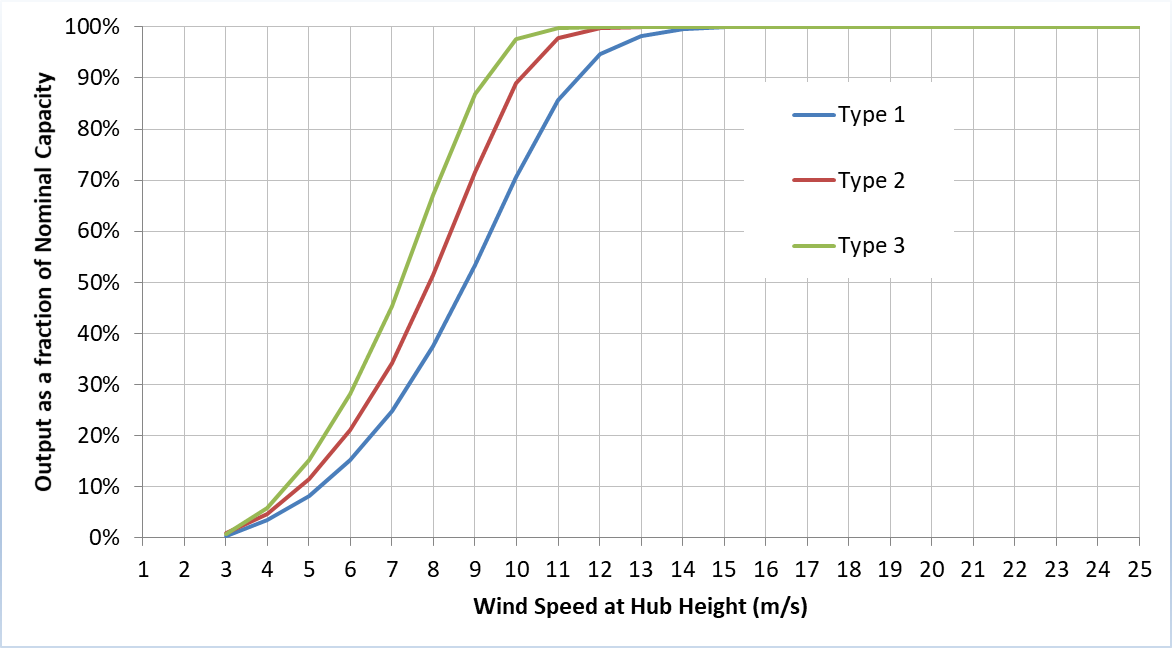
In the current version of US-REGEN, the candidate power curves are based on three composite power functions estimated by AWS Truepower (2012) rather than on specific turbine models. The three curves, shown in Figure 2-8, translate roughly to: (Type 1) a 2 MW turbine with 80m blades, i.e. specific power of ~400 W/m2; (Type 2) a 3 MW turbine with 112m blades, i.e. specific power of ~300 W/m2; and (Type 3) a 2.3 MW turbines with 116m blades, i.e. specific power of ~218 W/m2. These three composite power functions correspond to a generational evolution in the types of turbines deployed in the wind industry. Older capacity installations used turbines resembling the first type, while newer wind plants look increasingly like the third. Similarly, while older wind turbines were installed at hub heights of 80m or lower, new vintage turbines typically use towers of 100m or higher. REGEN on-shore wind output profiles for a given region / wind resource class vary by vintage based on an assumed mix of turbine type and hub height as detailed in Table 2‑3.
| Vintage | Hub Height | Turbine Type |
|---|---|---|
| Existing in 2015 | 80m | Mix across Types 1,2,3, varies by state/region |
| 2015-2020 | 100m | 50/50 mix between Type 2 and Type 3 |
| 2020-2025 | 50/50 mix between 100m and 120m | 50/50 mix between Type 2 and Type 3 |
| 2025-2030 | 50/50 mix between 100m and 120m | Type 3 |
| 2030-2050 | 120m | Type 3 |
For off-shore wind, the assumed technology through 2030 is a fixed platform 120m hub height turbine of Type 2, increasing to 140m in 2035. While turbines used for off-shore are typically much larger than those used for on-shore in terms of nominal output and swept area, their specific power is generally in the range described by the Type 2 composite, rather than the lowest on-shore specific power rating described by Type 3. EPRI has data for floating turbines, but the cost is significantly higher than for fixed platform turbines.
Solar Technologies
US-REGEN considers three types of central station solar PV technologies (fixed tilt crystalline silicon, single-axis tracking, and double-axis tracking), concentrated solar 'power tower' technology with endogenous determination of the amount of thermal storage, and fixed tilt rooftop solar PV. Note that the adoption of rooftop PV is modeled within the end-use model, and the resulting output profile is an exogenous input into the electric sector model.
Diffuse and direct irradiance are translated into output for a variety of solar photovoltaic technologies that are specified in terms of the orientation and tilt of the panels, which may be fixed or tracking the sun's position across the hours of the year. For fixed panels, we consider five distinct orientations (E, SE, S, SW, W) and five distinct tilt levels. The tilt levels are defined in terms of a proxy for optimal tilt, which is a function of latitude (roughly, the higher the latitude, the higher the optimal tilt angle). The five tilt levels are defined as 100%, 75%, 50%, 25%, and 0% of the optimal angle, where 0% refers to a flat horizontal panel (for which orientation is irrelevant). For tracking panels, we consider a single-axis configuration which has fixed tilt at the optimal angle and orientation matching the azimuth angle of the sun, and a double-axis configuration in which both tilt and orientation are adjusted so that the panel is always perpendicular to the incoming solar flux. For each grid cell and each hour, we calculate captured irradiance for a panel in all fixed and tracking combinations.
Captured irradiance has three components: direct, diffuse, and reflected. Each depends on the angle of the panel and the previously calculated direct and diffuse components of GHI. Direct captured irradiance is calculated based on the direct component of the incoming flux and the angle of the panel relative to the angle of incidence. Diffuse captured irradiance is calculated as a share of incoming diffuse component. Because the diffuse flux is coming down from all directions, the captured share is equal to 1 for a flat panel and less than 1 for tilted panels. The higher the tilt angle, the larger the "blind side" to the diffuse component, up to a hypothetical perfectly vertical panel, which would capture only half of diffuse irradiance. Finally, we assume that 20% of GHI is reflected (this fraction may vary depending on the albedo of the surface) and the direction is diffuse but with opposite sign. Hence a flat panel captures none of the reflected irradiance, and tilted panel would capture some, up to a hypothetical perfectly vertical panel, which would capture half of reflected irradiance.
While captured irradiance is calculated for all combinations of these variables, solar PV output profiles for the model are based on four distinct configurations: rooftop PV, fixed-tilt utility-scale PV, and single- and double-axis tracking PV. Rooftop solar PV is assumed to have fixed orientation and tilt, with a distribution over angles (see Table 2‑4). We assume that while rooftop installations attempt to configure panels in a south-facing orientation with optimal tilt, this is not always possible, resulting in a majority of installations being sub-optimal in one or both dimensions. We also represent fixed-tilt utility-scale PV, which is assumed to have south-facing orientation with optimal tilt. Single-axis and double-axis tracking are also represented as distinct configurations as described above.
| Flat | 25% | 50% | 75% | Optimal | |
|---|---|---|---|---|---|
| E | 0.01 | 0.01 | 0.01 | 0.01 | 0.06 |
| SE | 0.01 | 0.01 | 0.01 | 0.01 | 0.06 |
| S | 0.06 | 0.06 | 0.06 | 0.06 | 0.36 |
| SW | 0.01 | 0.01 | 0.01 | 0.01 | 0.06 |
| W | 0.01 | 0.01 | 0.01 | 0.01 | 0.06 |
Finally, captured energy at the panel is adjusted for temperature impacts on module efficiency, non-linear inverter losses, and a gross de-rating factor reflecting a range of factors not otherwise captured. Module temperature is estimated as a function of ambient temperature and incoming radiation. In full sun, module temperature can be 15-20 degrees C hotter than ambient temperature. Efficiency loss is calculated as a linear impact with coefficient 0.0045 per degree C delta between module temperature and an assumed rated temperature of 25 degrees C. This can result in impacts of roughly 10-15% in either direction for extreme hot or extreme cold conditions. Inverter are losses are assumed to be non-linear, such that losses are lowest in percentage terms when module output is highest but increase exponentially as output drops. This happens because a portion of inverter power loss remains constant regardless of input/output. The relationship between inverter loss and module output is calibrated such that losses at rated output are roughly 6%, increasing to roughly 26% at 10% of rated output. The implied average inverter efficiency is approximately 90%. In addition to inverter losses, we assume a gross de-rating factor of 0.9, i.e. additional 10% loss applied uniformly to the module profile, to reflect factors such as panel soiling, degradation, mismatch effects, and system availability.
Note that we currently assume inverter nominal AC capacity equal to solar module nominal DC capacity. In some installations, inverter capacity is sized lower than module capacity, which results in a higher nominal capacity factor (and potentially higher cost per nominal kW) because the ratio of nominal output to input is greater than 1. A smaller inverter may be used because losses on the DC side result in lower than nominal peak output, but it may also be used even when it implies curtailing or "clipping" some module output during peak conditions. Because these conditions occur only a few hours of the year, it may not be cost effective to size the inverter to capture fully all output. However, for utility-scale tracking configurations in high-quality resource areas, peak DC output as calculated by REGEN can exceed nominal output by as much as 15% (assuming nominal rating based on 1000 W/m2). In this situation, setting inverter capacity equal to nominal module capacity may be more suitable.
We also represent a concentrating solar power (CSP) technology with integrated thermal storage. The input profile for this technology is based on the DNI component only, as mirrors are assumed to track the sun's position and diffuse and reflected irradiance are not captured. Because of the high capital cost of CSP, it is assumed that this technology is only eligible for deployment in regions with sufficiently high resource quality, which we define as long-run average annual DNI of 2,600 kWh/m2. Figure 2‑11 shows the geographic area where this threshold is met, encompassing only seven states in the Southwest (CA, AZ, NV, NM, UT, CO, and TX). Because high-quality resource areas for CSP likely coincide with high-quality solar PV resource areas, the potential capacity constraint for solar within a given region / resource class is applied jointly across PV and CSP technologies.
(Class 1 is defined as > 2,800 kWh/m2, Class 2 as > 2,600 kWh/m2)
Distributed Energy Resources
The model begins with estimates of the total technical potential for rooftop solar PV capacity from NREL (2016) and solar resource profiles from the MERRA-2 reanalysis dataset as described in the Renewable Resources page. The initial economic potential is then calculated based on current market conditions, which helps to calibrate the model to historical uptake data. For each structural class, the model determines an average payback period for future vintages based on projections of retail prices from the electric sector model, capital costs of new systems, and applicable incentives (e.g., investment tax credits). These discounted cash-flow calculations are performed across structural classes to evaluate the variation in economic competitiveness of DER across heterogeneous customers. Structural classes are based on buildings data from the end-use model, which includes exogenous projections for the number of housing units and commercial square footage by building vintage, building size, and model region over time. Maximum technical potential is allocated based on the existing housing stock and commercial floor space and is assumed to scale over time with net additions. Policies currently represented in the model include the federal investment tax credit and California's residential rooftop PV mandates.
Cost markups for behind-the-meter solar PV for residential and commercial installations are based on NREL's 2018 Annual Technology Baseline (ATB) Mid scenario, which are 82% and 35% respectively in 2030.[4] Markups for new construction vis-à-vis retrofits are based on values reported by Lawrence Berkeley National Laboratory (2018). Capital costs include a $250/kWAC hookup charge.
After the cost and value of rooftop installations are used to calculate the payback period from residential and commercial consumer perspectives, the potential DER adoption rate (i.e., economic potential as a fraction of total potential) is calculated as an exponential function of the payback period. The function is calibrated so that there is 10% adoption with a 12-year payback period and 100% adoption with immediate payback. Curves are calculated across model regions accounting for variation of payback periods around the average. Similar to other sectors in the end-use model, the DER retail market model calculates potential adoption rates based on economic conditions in each period but assumes that actual deployment is based on a lagged diffusion process. The model converges from the initial potential rate toward the calculated rate with a 0.5 step length per period to avoid unrealistic increases for specific regions and calculates new additions based on convergence toward potential adoption at 6% per year for residential buildings and 3% per year for commercial buildings.[5]
Capacity from the DER retail market model and other end-use model outputs are sent to the electric sector model. The updated retail electricity prices from the electric sector model are then used as inputs for the next iteration of the DER retail market model. The models iterate until prices converge.
Hydro and Geothermal
New additions of hydroelectric power are limited to planned or underway projects through 2015.
New additions of geothermal power capacity are constrained based on estimates of discovered and undiscovered conventional sites in the western regions by NREL (2007). Total new potential additions amount to roughly 30 GW by 2050. US-REGEN also assumes an improving capacity factor for geothermal power over time as a result of technical progress, from roughly 50% today to roughly 80% by 2050. The assumed capital costs for new geothermal capacity are around $5000/kW (declines slightly over time). The assumed cost level / potential quantity corresponds to the "lowest rung" of the supply curve, i.e. most accessible geothermal resources.
Other Assumptions
There are a variety of constraints placed on new additions of individual technologies at both the regional and national level in the base scenario. These constraints are summarized here and in the subsequent table.
There are no limitations on new additions of fossil generation in the base case scenario. Limitations may be applied in specific scenarios.
New additions of biomass co-firing capacity are limited to 5 GW per region per time period (in some scenarios this constraint is relaxed).
New additions of nuclear are constrained at the national level.
New additions of "brownfield" nuclear are constrained at the regional level based on available existing or pre-developed nuclear sites in each state.
Some states have regulations that effectively prohibit the construction of new nuclear units, including California, Kentucky, and West Virginia, to name a few. US-REGEN uses data from the National Conference of State Legislators to inform which states prohibit new nuclear units.[6]
This percentage was determined based on input from the EPRI Nuclear Sector's long-term operation experts. It can be varied in certain scenarios, for example in some cases all licenses are assumed to end at 60 years. No additional cost is incurred to extend the unit's life. ↩︎
There was no existing offshore wind capacity at the end of 2015. ↩︎
Both coal and gas can be marginal generators depending on the region and time of day. Even in 2007, when gas prices were very high, not all coal units were in the money all the time. ↩︎
Available at: https://atb-archive.nrel.gov/electricity/2018/index.html. ↩︎
The commercial diffusion rate is lower than the residential rate to reflect additional heterogeneity, market barriers, and prevalence of non-owner occupied buildings. ↩︎
See https://www.ncsl.org/research/environment-and-natural-resources/states-restrictions-on-new-nuclear-power-facility.aspx. ↩︎